Authors: brilliant predecessors in my interpretation. Composed by VVvv. Translated by Google translate, ran by VVvv.
Thanks are given to professors Jiri Bicak and Michal Krizek.
Version Tenerife – Miraverde, June 22, 2021
Translated December 8, 2022
In Looking into Universe, in four parts, in four stages, the implications of using a model of curved space with constant curvature to represent the space of the Universe as a whole are elaborated. In the first part, the consequences for light propagation and observation in such a space are explained. In the second part, the consequences of expanding such a space are elaborated. In the third part, the consequences of a broader understanding of the space-time modeled in this way are presented, and in the fourth part, the consequences of the closed and open space model are compared.
In this second part, we follow up on the consequences presented in the first part, which warn us that in a closed space with constant curvature, simultaneous multiple observations of the same objects from different sides cannot be avoided. The light spreads in it in a straight direction, which we replace with SUBSTITUTIVE CIRCLES when looking from the outside.
The coordinate in the directions of observation along the arc can be expressed as z=R·φ, where R is the radius of curvature and the angle φ is measured in the arc measure with the origin at the point of observation. Then, for fixed points (φ constant) on a circle that changes its radius R over time, we can express the temporal change of the observed distance as dz/dt= dR/dt·φ, and by marking dR/dt with the symbol ΔV0, as ΔV=ΔV0·φ. So the increasing rate of receding of observed objects with distance φ (dz/dt=ΔV as a function of φ) for expanding circles (dR/dt=ΔV0>0) is an inherent property of the model.
This is an effect that must be equally observed from all points of space, as we consider such observations to be valued equally. Therefore, even the expansion of space, which I call ECSTASY here (dR/dt= ΔV0 >0), must be the same in all points of space, because there is no EXCEPTIONAL place in it where anything could happen differently.
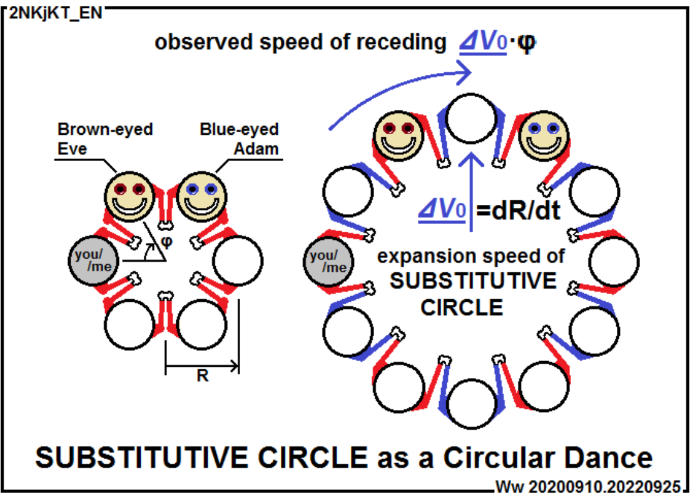
Let´s imagine such a process of uniform expansion of space and thus the expansion of the SUBSTITUTIVE CIRCLES that represent our observations in it, for example, as suggested by the figure SUBSTITUTIVE CIRCLE as a Circular Dance [2NKjKT_EN]:
In the left part, we see schematically as if you, or I, were dancing in a circle next to brown-eyed Eve and blue-eyed Adam, all in a red suit. The radius of the circle R is indicated here, as well as how the angle φ is measured by us.
On the right part, other blue-clad dancers join the dance spatially evenly. It is easy to see from the sketch that Adam will move away from us faster than Eve (and we will also move away from Adam faster than Eve). Or to put it another way: The more distant dancers will automatically move away from us faster than the closer ones, despite the fact that their position angle φ on the circle does not change. The increase in the radius of the circle is indicated, i.e. the expansion of the SUBSTITUTIVE CIRCLE. For the expansion speed dR/dt =ΔV0, the observed receding speed along the arc is ΔV = ΔV0 ·φ.
Since the φ of our observation can grow indefinitely, then for a sufficiently large φ the observed velocity of retreat will reach the limiting value of the speed of light propagation c, i.e. ΔV =c. This is an inherent effect of the model I call the LIGHT BARRIER of the Universe (SBV). The closest equivalent established terms in physics are the cosmological horizon or the Horizon of the Universe (HV), or the limit of the observable Universe from the observer’s point of view.
Because light travels to us at speed c, this distance corresponds to a certain interval of the passage of time at our point of observation, which we will call the AGE of UNIVERSE (VV). So then HV≡ SBV= c·VV. For the expansion of
the Universe by ECSTASY at a speed of ΔV0, we can then call the Observable SIZE of UNIVERSE RPV as the distance to which the space was carried away by ECSTASY from us in all directions during the AGE of UNIVERSE VV. This gives us RPV= ΔV0·VV.
However, since the most distant object observed along a circular arc can never lie further than on the opposite side of the circle, i.e. at a distance of φ= π from the observer, then the corresponding SIZE of UNIVERSE comes out as Rv= R·π.
Another consequence of observation in such a model concerns the so-called Twin Paradox in physics, which corresponds to two opposing views on observation in the space of the Universe. One that Albert Einstein created for us, and which rules out the existence of any EXCEPTIONAL frame of reference in the Universe. All reference systems to which we formulate physical laws must be equal to each other, none must be EXCEPTIONAL. And the second one, which Hendrik Lorentz made for us, and in which it is required that there should be at least one UNIQUE reference system for observers in the Universe to which our IDEA of relativity could be related.
The considered model separates the local system, in which each of us subjectively looks into the Universe, from the system of the entire objective curved space with constant curvature.
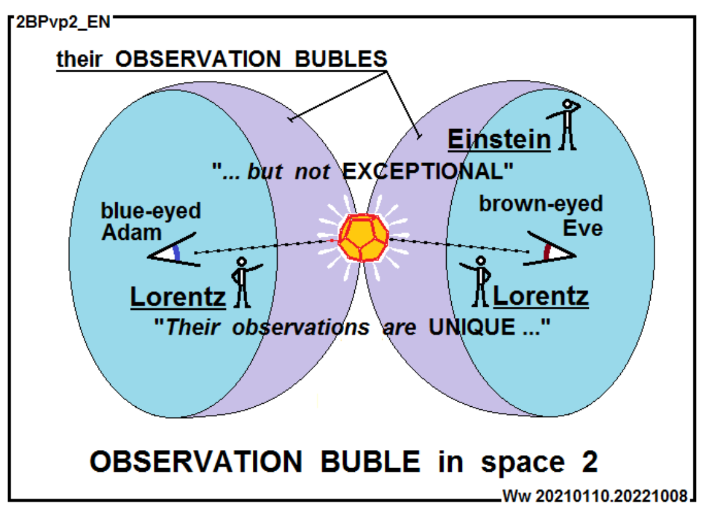
The picture OBSERVATION BUBBLE in space 2 [2BPvp2_EN] shows, as an example, how brown-eyed Eve and blue-eyed Adam observe one and the same object, perhaps a jewel, at the common point of their OBSERVATION BUBBLE, onto which they seem to project all observations. For both observers the observation is UNIQUE according to Lorenz, but at the same time neither of them is EXCEPTIONAL according to Einstein. Our model does not create any Twin Paradox for us
Another consequence that the model draws our attention to is that we observe distances between objects in the distant Universe greater than they are in space. This distortion of our observation is the reason why there seems to be a lack of gravity in the distant Universe, as if there is a GRAVITY DEFICIT. This is made visible, for example, by the figure Distortion of Observed Distances [2ZpV_EN], which combines all 3 sketches from the figure STARS and LIGHT PROPAGATION G [2phG_EN] from the first part of this file into one:
But we will simplify the situation by considering the drawn stars “S1“, “S2” and “S3” as if they existed at the same time. Therefore, we stop the flow of time for a while, so we freeze their positions in space-time. Although, for example, the light from S2 flew to us observers at point “P” along an arc at a distance of L2=R·π/2 and from S3 at a distance twice L3=R·π (R is the radius of curvature of space), and thus we are actually observing the star S3 in a double past than the S2 star, we will consider their positions unchanging in space by freezing the passage of time.
From the observer at point P, star S2 is distant V2=R·√2 and star S3 V3=2·R, resulting in their actual mutual distance marked in blue V23=(2-√2)•R ≈0,5858·R. But we, as observers in P, observe the star S2 in its apparent position Z2 from us at a distance L2=R·π/2 in a direction 45° deviated from the P-S2 connecting line. And we observe the star S3 in its apparent position Z3 from us at a distance L3=R·π in a direction 90° deviated from the same connecting line. Their mutual angle in our observation will therefore be 45°.
According to the cosine theorem, the square of their mutual distance observed by us is L232=L22+L32-2·L2·L3·cos45°=R2·π2·(5/4-1/√2)≈ 5,35814·R2, and their observed distance L23≈ 2,3148·R versus the actual distance V23≈0,5858·R, so L23/V23≈ 3,95. The distorted L23 distance is almost four times larger (!) than the true V23 distance, and thus the corresponding mutual gravitational effect would be almost sixteen times weaker (!!) for this situation.
Similarly, if we consider that the star S1 is close to us (L1≈V1≈0) and thus the influence of the curvature of space on its observation is still negligible, we calculate the observed distortion and the actual distance between S1 and S2 as L12≡L2= R·π/2≈ 1,5708·R and V12≡V2= R·√2≈ 1,4142·R, so L12/V12≈ 1,11. Due to the distortion, the observed distance also increased, but only slightly by approximately 11% compared to the actual distance.
Although we consider the special case of observing objects from us in a straight line behind one another, and by freezing the passage of time we limit the validity to their mutual distances significantly smaller than the radius of curvature of space, and the speed of their changes in position in space significantly smaller than the speed of light, we have shown how
the model predicts an amplifying effect of increasing the mutual distances of observed objects with their increasing distance from us.
If we consider such large distortions of mutual distances between distant objects observed in the Universe, as the described model predicts, we cannot avoid considering a large GRAVITY DEFICIT between them.
This could point to a hitherto misinterpreted curvature effect, which may have required so-called dark matter to be introduced to compensate for the missing gravity. That is, some invisible mass whose inertial effects we do not observe, only its gravitational effects. Its amount is estimated in the quote https://en.wikipedia.org/wiki/Dark_matter: “Dark matter is a form of matter thought to account for approximately 85% of the matter in the universe“. That is such a large amount that only 15% (!!!) of observable “light matter” would be left in the Universe.
In conclusion, the second challenge is presented: to determine the consequence of the distortion of the mutual distances that the model predicts, in specific situations, and thus also to determine the size of the GRAVITY DEFICIT. It would be verified to what extent the observed lack of gravity is due to the predicted distortion of the observed mutual distances.