Authors: brilliant predecessors in my interpretation.
Composed by VVvv. Translated by Google translate, ran by VVvv.
Thanks are given to professors Jiri Bicak and Michal Krizek.
Netherlands – Alkmaar version, July 26, 2021
Translated August 17, 2021
We consider here the space-time shown schematically in the figure Curved versus non-curved space-time [0ZvNCP_EN], which at each point “P” appears to the observer as if time information (t) was coming to him from all sides, i.e. in the spherical coordinate system (r, φ, ψ) from any combination of directional angles φ and ψ, so that -r=c•t, where c denotes the speed of incoming information. That is to say, from greater and greater distance and past.
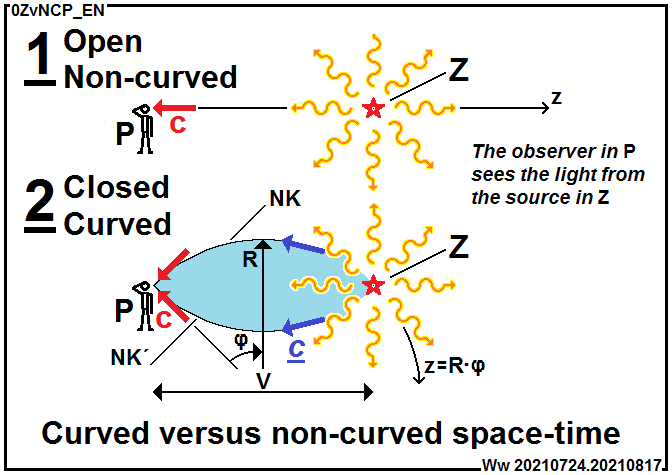
And in which from each source “Z” at any point the time information emerges spherically in all directions as r=c•t, where c denotes the speed of outgoing information.
In such a model of non-curved space 1, the propagation (c=c) of information appears geometrically along a straight line from point “Z” to point “P“, i.e. along the “z” axis in the Cartesian coordinate system (x, y, z).
In curved space 2, information propagation is modeled as following an arc (z=R•φ) spatially with a constant radius of curvature “R“. Any two points “V” away from each other (for 0<V<2R) can be geometrically connected in a plane by two arcs of radius R (= we can inset two circles “NK” and “NK´” of radius R into two points in plane). However, we can three-dimensionally connect the two points with the surface of a shape called “rugball“, colored light blue in the image, which is created by rotating the arc “NK” (or “NK´“) around the line Z-P.
Such a model of self-contained space-time with spatially constant curvature applied to our looking into the Universe then predicts the consequence that we cannot avoid observing individual objects in multiple directions at once. The individual views, which we can mistakenly consider to be different objects, are arranged in the sky in a circle, the diameter of which increases with the increasing distance to the observed object, and whose center lies in the direction of the P-Z line, where the observed object seems to be hiding from us in space-time.
First part Looking into Universe.1 describes in detail this consequence of such a model on the propagation of light through curved space and on the observation of objects in such space. It further encourages how such objects or their groups that we observe twice or more in different directions could be searched for in the sky accordingly. But it is a much broader invitation than some failed attempts at the same thing in the past. If such multiple observations predicted by the model could be found in the Universe, then it would undoubtedly be an encouragement that even such a simple model could be useful for our insight into the space of the Universe as a whole.
